next up previous
Next: Relation to the Wigner Up: The case of nearly-diagonal Previous: The case of nearly-diagonal

Formulation and Proof of the Lemma

Let us start with Hamiltonian (4) without off-diagonal terms, so that $ B(\textbf{q},\textbf{q}_1)\equiv 0$ . This is a typical Hamiltonian for linear waves in weakly inhomogeneous media [10] expressed in terms of Fourier amplitudes $ a_{\textbf{q}}$ and $ a^*_{\textbf{q}_1}$ as
$\displaystyle H=\int\Omega (\textbf{q},\textbf{q}_1)a_\textbf{q}a^*_{\textbf{q}_1}d\textbf{q}d\textbf{q}_1,$     (21)

with a Hermitian kernel $ \Omega (\textbf{q}_1,\textbf{q})=\Omega ^*(\textbf{q},\textbf{q}_1)$ , which is strongly peaked at $ \textbf{q}-\textbf{q}_1=0$ ($ \Omega $ has a finite support around $ \textbf{q}\simeq\textbf{q}_1$ ). Therefore, we subsequently assume that there is a small parameter $ \varepsilon $ for which
$\displaystyle \Omega (\textbf{q}_1-\textbf{q}) = 0$     (22)

when $ \vert\textbf{q}-\textbf{q}_1\vert>\varepsilon $ . A particular choice
$\displaystyle \Omega(\textbf{q}_1,\textbf{q})=\omega(\textbf{q}_1)\delta(\textbf{q}-\textbf{q}_1),$      

leads to the familiar form of the Hamiltonian (2).
The equation of motion for $ a_\textbf{k}$ is
$\displaystyle i\dot{a}_\textbf{k}= \frac{\delta H}{\delta a_\textbf{k}^*} = \int \Omega _{\textbf{q}\textbf{k}}a_\textbf{q}d\textbf{q}.$     (23)

Lemma 1   Consider the Hamiltonian (23) with $ \Omega (\textbf{q},\textbf{q}_1)$ being a peaked function of $ (\textbf{q}-\textbf{q}_1)$ (satisfying (24)) and a smooth function of $ (\textbf{q}+\textbf{q}_1)$ . Then there exist a near-canonical change of variables $ \hat{a}_\textbf{k}\rightarrow\check{a}_{\textbf{k}\textbf{x}}$ such that in the new variables the equation of motion can be written in the Hamiltonian form

$\displaystyle i\frac{\partial}{\partial t}{\check{a}_{\textbf{k}\textbf{x}}} = \frac{\delta H_f}{\delta \check{a}^*_{\textbf{k}\textbf{x}}}, $

with the filtered Hamiltonian in a canonical form
$\displaystyle H_f=\int \check{a}_{\textbf{k}\textbf{x}} [\omega _{\textbf{k}\te... ...k}\textbf{x}},\cdot\}]\check{a}_{\textbf{k}\textbf{x}}^*d\textbf{k}d\textbf{x},$     (24)

where $ \omega _{\textbf{k}\textbf{x}}$ is the position dependent frequency related to $ \Omega (\textbf{q},\textbf{q}_1)$ via the Wigner transform
$\displaystyle \omega _{\textbf{k}\textbf{x}}=\int e^{i\textbf{m}\cdot\textbf{x}}\Omega (\textbf{k}-{\textbf{m}}/2,\textbf{k}+{\textbf{m}}/2)~d\textbf{m}.$     (25)

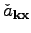
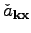
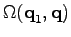
Proof. In order to obtain the new variables $ \check{a}_{\textbf{k}\textbf{x}}$ , we first make a window transform via (17), which is then followed by a near-identical transformation to the new variables $ \check{a}_{\textbf{k}\textbf{x}}$ . The idea of the proof is to use the peakness of the kernel $ \Omega(\textbf{q}_1,\textbf{q})$ . We make a Taylor expansion around the peak and then by neglecting the higher order terms we obtain the desired result.

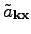We make a window transform from $ a_\textbf{k}$ to $ \tilde{a}_{\textbf{k}\textbf{x}}$ using Eq. (20). Differentiating Eq. (20) with respect to time, using the Eq. (25), and applying the inverse formula (21) yield

$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}$ $\displaystyle =$ $\displaystyle \left(\frac{1}{\varepsilon^*}\right)^d\int \hat{f}(\vert\textbf{k... ...\textbf{x}}\Omega _{\textbf{q}\textbf{q}_1}a_\textbf{q}d\textbf{q}d\textbf{q}_1$  
  $\displaystyle =$ $\displaystyle \left(\frac{1}{\sqrt{\pi}}\right)^d\int \hat{f}(\vert\textbf{k}-\... ..._1}\tilde{a}_{\textbf{q}\textbf{x}_1} {d\textbf{q}d\textbf{q}_1 d\textbf{x}_1}.$ (26)

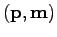
Let us change variables from $ (\textbf{q},\textbf{q}_1)$ to $ (\textbf{p},\textbf{m})$ as
$\displaystyle \textbf{q}$ $\displaystyle =$ $\displaystyle \textbf{p}-{\textbf{m}}/2,$ (27)
$\displaystyle \textbf{q}_1$ $\displaystyle =$ $\displaystyle \textbf{p}+{\textbf{m}}/2.$ (28)

Below it will be convenient to use
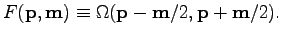$\displaystyle F(\textbf{p},\textbf{m})\equiv\Omega (\textbf{p}-{\textbf{m}}/2,\textbf{p}+{\textbf{m}}/2).$     (29)

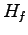
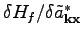
Next, we will approximate the RHS of Eq. (28) by a variation of some filtered Hamiltonian $ H_f$ , i.e., by $ \delta H_f/\delta\tilde{a}_{\textbf{k}\textbf{x}}^*$ . We can rewrite Eq. (28) as
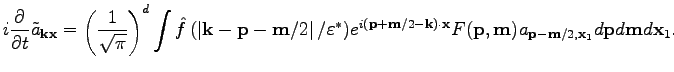$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}=\l... ... a_{\textbf{p}-{\textbf{m}}/2,\textbf{x}_1}d\textbf{p}d\textbf{m}d\textbf{x}_1.$     (30)

Let us make another change of variables $ \textbf{p}\rightarrow \textbf{p}+{\textbf{m}}/2$
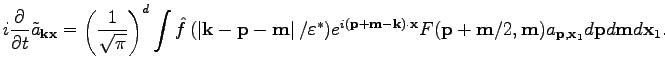$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}=\l... ...}/2,\textbf{m}) a_{\textbf{p},\textbf{x}_1}d\textbf{p}d\textbf{m}d\textbf{x}_1.$     (31)

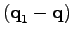
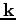
In order to simplify Eq. (33), we are going to use the fact that $ \Omega _{\textbf{q}\textbf{q}_1}$ and $ \hat{f}(\textbf{k})$ are peaked functions of $ (\textbf{q}_1-\textbf{q})$ and $ \textbf{k}$ , respectively, and fast decaying at infinity. We also keep only first order terms in spatial derivatives, neglecting second and higher order terms. Then, we could write
$\displaystyle \hat{f}(\vert\textbf{k}-\textbf{p}-\textbf{m}\vert/\varepsilon^*)... ...m}\cdot\nabla_\textbf{p}\hat{f}(\vert\textbf{k}-\textbf{p}\vert/\varepsilon^*)+$h.o.t.     (32)

Similarly, we obtain
$\displaystyle F(\textbf{p}+{\textbf{m}}/2,\textbf{m})=F(\textbf{k}+\textbf{p}-\... ...bf{p}-\textbf{k}+{\textbf{m}}/2)\cdot\nabla_\textbf{k}F(\textbf{k},\textbf{m})+$h.o.t.     (33)

where h.o.t. denotes higher order terms. Now, we substitute the expansions (34) and (35) into Eq. (32), and after ignoring higher order terms, we obtain
$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}=$   $\displaystyle \left(\frac{1}{\sqrt{\pi}}\right)^d$  
    $\displaystyle \times \int\Big(\hat{f}(\vert\textbf{k}-\textbf{p}\vert/\varepsil... ...\vert/\varepsilon^*)\Big)e^{i(\textbf{p}+\textbf{m}-\textbf{k})\cdot\textbf{x}}$  
    $\displaystyle \times\big(F(\textbf{k},\textbf{m})+ (\textbf{p}-\textbf{k}+{\tex... ...m})\big)\tilde{a}_{\textbf{p},\textbf{x}_1}d\textbf{p}d\textbf{m}d\textbf{x}_1.$  

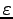
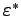
Note that, here we have an expansion with two different small parameters $ \varepsilon $ and $ \varepsilon^*$ , which obey Eq. (18).

In Appendix A, we show how to simplify the RHS of Eq. (36). As a result of this simplification, we obtain

$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}$ $\displaystyle =$ $\displaystyle \omega _{\textbf{k}\textbf{x}}\tilde{a}_{\textbf{k}\textbf{x}}-\t... ...textbf{x}}(\textbf{x}\cdot\nabla_{\textbf{x}}\tilde{a}_{\textbf{k}\textbf{x}})}$  
    $\displaystyle +i/2(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\... ...{x}}(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\tilde{a}_{\textbf{k}\textbf{x}}}.$ (34)

This equation can be written in the Hamiltonian form
$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}=\frac{\delta H_f}{\delta\tilde{a}_{\textbf{k}\textbf{x}}^*},$      

where the filtered Hamiltonian takes form
$\displaystyle H_f$ $\displaystyle =$ $\displaystyle \int\Big((\omega _{\textbf{k}\textbf{x}}-\textbf{x}\cdot\nabla_\t... ...{x}}^*(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\tilde{a}_{\textbf{k}\textbf{x}}$  
    $\displaystyle ~~~~+i\tilde{a}_{\textbf{k}\textbf{x}}^*(\nabla_\textbf{x}\omega ... ... _{\textbf{k}\textbf{x}}\cdot\nabla_\textbf{x}\tilde{a}_{\textbf{k}\textbf{x}}+$  
    $\displaystyle ~~~~~~~ (\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf... ...tbf{k}\textbf{x}}\tilde{a}_{\textbf{k}\textbf{x}}) \Big)d\textbf{k}d\textbf{x}.$ (35)

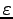
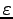
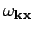
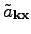
Now, we will use the general method of the WKB approximation. We will only keep the terms, which are of the first order in a small parameter $ \varepsilon $ . In our case, the small parameter $ \varepsilon $ characterizes the rate of spatial change of the position dependent frequency $ \omega _{\textbf{k}\textbf{x}}$ and the dynamical variable $ \tilde{a}_{\textbf{k}\textbf{x}}$ . To apply the WKB approximation to Eq. 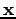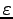(37), we neglect the terms that have two derivatives with respect to $ \textbf{x}$ (underlined) because each spatial derivative is of the order $ \varepsilon $ small. As a result, we obtain the following equation of motion
$\displaystyle i\frac{\partial}{\partial t}{\tilde{a}_{\textbf{k}\textbf{x}}}=\o... ...abla_\textbf{x})\omega _{\textbf{k}\textbf{x}}\tilde{a}_{\textbf{k}\textbf{x}}.$     (36)

However, Eq. (39) becomes non-Hamiltonian. Indeed, the corresponding functional
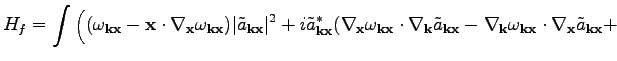$\displaystyle H_f=\int\Big((\omega _{\textbf{k}\textbf{x}}-\textbf{x}\cdot\nabl... ... _{\textbf{k}\textbf{x}}\cdot\nabla_\textbf{x}\tilde{a}_{\textbf{k}\textbf{x}}+$      
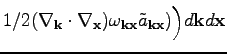$\displaystyle 1/2(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}}\tilde{a}_{\textbf{k}\textbf{x}}) \Big)d\textbf{k}d\textbf{x}$     (37)

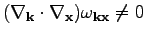
is not self-conjugate if $ (\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}}\neq 0$ . Therefore, in order to obtain canonical equations of motion, another near-canonical change of variables needs to be performed
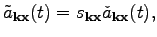$\displaystyle \tilde{a}_{\textbf{k}\textbf{x}}(t)=s_{\textbf{k}\textbf{x}}\check{a}_{\textbf{k}\textbf{x}}(t),$     (38)

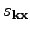
where $ s_{\textbf{k}\textbf{x}}$ is some time-independent function to be determined below. Note that transformation (41) is canonical if and only if
$\displaystyle \vert s_{\textbf{k}\textbf{x}}\vert^2=1.$      

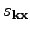
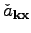
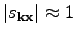
Therefore, we need to find such $ s_{\textbf{k}\textbf{x}}$ that the system becomes Hamiltonian in terms of new variables $ \check{a}_{\textbf{k}\textbf{x}}$ and the transformation (41) is near-canonical, i.e., $ \vert s_{\textbf{k}\textbf{x}}\vert\approx 1$ . We substitute Eq. (41) into Eq. (39) to obtain
$\displaystyle is_{\textbf{k}\textbf{x}}\frac{\partial}{\partial t}\check{a}_{\textbf{k}\textbf{x}}$ $\displaystyle =$ $\displaystyle s_{\textbf{k}\textbf{x}}\big[(\omega _{\textbf{k}\textbf{x}}-\tex... ...textbf{k}\textbf{x}}\cdot\nabla_\textbf{x}\check{a}_{\textbf{k}\textbf{x}}\big]$  
    $\displaystyle +\check{a}_{\textbf{k}\textbf{x}}[i\nabla_\textbf{x}\omega _{\tex... ...\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}}s_{\textbf{k}\textbf{x}}].$  

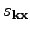
If we find $ s_{\textbf{k}\textbf{x}}$ that satisfies the equation
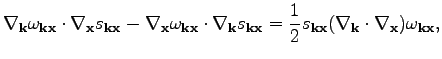$\displaystyle \nabla_\textbf{k}\omega _{\textbf{k}\textbf{x}}\cdot\nabla_\textb... ...tbf{x}}(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}},$     (39)

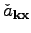
then the equation of motion in the new variables $ \check{a}_{\textbf{k}\textbf{x}}$ takes the canonical form
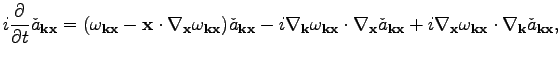$\displaystyle i\frac{\partial}{\partial t}{\check{a}_{\textbf{k}\textbf{x}}}=(\... ... _{\textbf{k}\textbf{x}}\cdot\nabla_\textbf{k}\check{a}_{\textbf{k}\textbf{x}},$     (40)

with the corresponding Hamiltonian (26). In order to find a solution of Eq. (42), we make a change of variables
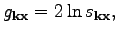$\displaystyle g_{\textbf{k}\textbf{x}}=2\ln s_{\textbf{k}\textbf{x}},$     (41)

to obtain
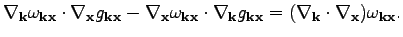$\displaystyle \nabla_\textbf{k}\omega _{\textbf{k}\textbf{x}}\cdot\nabla_\textb... ...bf{x}}=(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}}.$     (42)

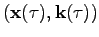
We find the solution of Eq. (45) using the method of characteristics. The characteristics $ (\textbf{x}(\tau),\textbf{k}(\tau))$ are given by the following equations
$\displaystyle \frac{d\textbf{x}}{d\tau}$ $\displaystyle =$ $\displaystyle \nabla_\textbf{k}\omega _{\textbf{k}\textbf{x}},$ (43)
$\displaystyle \frac{d\textbf{k}}{d\tau}$ $\displaystyle =$ $\displaystyle -\nabla_\textbf{x}\omega _{\textbf{k}\textbf{x}},$  

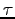
where $ \tau$ is a parameter along the characteristics. Physically these characteristics correspond to the trajectories (rays) of WKB wavepackets in the $ (\textbf{x},\textbf{k})$ space. The solution of Eq. (45) is given by
$\displaystyle g(\textbf{x}(\tau),\textbf{k}(\tau))=\int_0^\tau(\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}}~d\tau'.$      

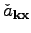
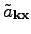
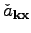
Now, we use Eq. (44) and then Eq. (41) in order to obtain the new variable $ \check{a}_{\textbf{k}\textbf{x}}$ out of the Gabor variable $ \tilde{a}_{\textbf{k}\textbf{x}}$ . The dynamics of the new variable $ \check{a}_{\textbf{k}\textbf{x}}$ is described by the filtered Hamiltonian (26). $ \qedsymbol$

Note that for the special case $ (\nabla_\textbf{k}\cdot\nabla_\textbf{x})\omega _{\textbf{k}\textbf{x}}=0$ , the Gabor variables $ \tilde{a}_{\textbf{k}\textbf{x}}$ provide a Hamiltonian structure (26). Then, in this special case, there is no need in performing the second transformation (41). We will see below in the examples that this observation may significantly simplify the applications of the Lemma.

To describe the statistical properties of spectral energy transfer in the systems, it is convenient to define a position dependent wave action as

$\displaystyle \check{n}_{\textbf{k}\textbf{x}}\equiv \langle\vert\check{a}_{\textbf{k}\textbf{x}}\vert^2\rangle .$     (44)

Using this definition, one obtains from (26) the familiar form of the kinetic equation (sometimes called radiative balance equation) in a weakly inhomogeneous media, by using the Eq. (43). The resulting equation for the time evolution of $ \check{n}_{\textbf{k}\textbf{x}}$ is the Equation (6) of the introduction. This is the so-called waveaction transport which is typical for WKB systems.

next up previous
Next: Relation to the Wigner Up: The case of nearly-diagonal Previous: The case of nearly-diagonal
Dr Yuri V Lvov 2008-07-08