Conformal canonical variables

The basic set of equations describing a two-dimensional potential flow of an ideal incompressible fluid with a free surface in a gravity field fluid is the following one:

$$\phi_{xx} + \phi_{zz} = 0 \hspace{1cm} (\phi_z\to 0, z\to -\infty), \cr \eta_t + \eta_x\phi_x$$

here $\eta(x,t)$ - is the shape of a surface, $\phi(x,z,t)$ - is a potential function of the flow and $g$ - is a gravitational constant. As was shown by Zakharov in[1], potential on the surface $\psi(x,t) = \phi(x,z,t)\bigg\vert _{z=\eta}$ and $\eta(x,t)$ are canonically conjugated, and their Fourier transforms satisfy the equations

$$\frac{\partial \psi_k}{\partial t} = -\frac{\delta \mbox{$\ca... ...}{\partial t} = \frac{\delta \mbox{$\cal H$}}{\delta \psi_k^*}.$$

Here $\mbox{$\cal H$}=K+U$ is the total energy of the fluid with the following kinetic and potential energy terms:

$$K = \frac{1}{2}\int\!dx\!\int_{-\infty}^\eta\,v^2\!dz \hspace{1cm} U = \frac{g}{2}\int \eta^2\!dx$$

A Hamiltonian can be expanded in an infinite series in powers of a characteristic wave steepness $k\eta_k <\!< 1$ (see[,]) by using iterative procedure. All terms up to the fifth order of this series contribute to the amplitude of five-wave interaction.

Here we prefer to do that performing first a certain canonical transformation from the variables $\psi$, $\eta$ to the new canonical variables.

Let us perform, following Kuznetsov, Spector and Zakharov [8], a conformal mapping of the domain $z < \eta(x,t)$ to the lower half-plane of the complex variable $w = u + iv, -\infty<u<\infty, -\infty<v<0$. The shape of the surface is parametrized by two functions

$$z(u,t), \hspace{0.5cm} x(u,t)$$

which are connected by the Hilbert transformation

$$x(u,t) = u - \hat H(z(u,t))\cr \hat H(f(u))$$

We introduce also the complex velocity potential

$$\Phi(w,t) = \Psi(u,v,t)+i\Theta(u,v,t)$$

On the surface ($v=0$)

$$\Theta(u,0,t) = \hat H(\Psi(u,0,t)), \cr$$

New canonical variables can be obtained using variational principle for the action. With old variables action is

$$S = \int dt \{ \int \psi(x,t)\eta_t(x,t)dx - \mbox{$\cal H$}\}.$$

After conformal mapping[8] it acquires the form:

$$S = \int L dt, \cr L$$

Lagrangian function $L$ can be rewritten as

$$L = \int \{z_t(\Psi x_u - \hat H(\Psi z_u)) + \frac{1}{2}\Psi \hat H\Psi_u - \frac{g}{2} y^2 x_u \}du.$$

and the new canonical variables are $z(u,t)$ and $\mbox{$\cal P$}(u,t) = \Psi x_u - \hat H(\Psi z_u)$. $\Psi$ can be easily inverted as

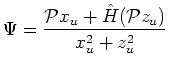$$\Psi = \frac{\mbox{$\cal P$}x_u + \hat H(\mbox{$\cal P$}z_u)}{x_u^2+z_u^2}$$ (2.1)

and the Hamiltonian of the system is

$$\mbox{$\cal H$}= \frac{1}{2} \int \{g z^2 x_u - \Psi \hat H\Psi_u\} du$$

where $\Psi$ is equal to (2.1). The equations of motion can be written in the explicit Hamiltonian form which includes integral Hilbert's operator:

$$\frac{\partial \mbox{$\cal P$}}{\partial t} = -\frac{\delta \mbox... ...\partial z}{\partial t} = \frac{\delta \mbox{$\cal H$}}{\delta \mbox{$\cal P$}}$$