Introduction

In this article we study interaction of gravity waves propagating in one direction on the surface of ideal fluid of infinite depth. The problem is of a big theoretical and practical importance. It is known from the experiment that the distribution function of wave energy even in the active zone of a storm is almost one-dimensional in the energy-containing domain. Even more this is correct for the ``swell'' far away from the active zone. That is the point of common belief that the main mechanism of wave interaction is the four-wave scattering, satisfying the following resonant conditions

$$\omega_{k} + \omega_{k_1} = \omega_{k_2} + \omega_{k_3} \cr \vec k + \vec k_1$$ (1.1)

here $\vec k_i$ are the wave vectors of the interacting waves, and $\omega_k = \sqrt{gk}$ - the dispersion law. The corresponding effective Hamiltonian has the form

$$\mbox{$\cal H$} = \int \omega_k a_k a_k^* dk+\cr$$ (1.2)

($a_k$ are complex amplitudes of propagating waves [1],[2],[3]) and the corresponding kinetic equation is

$$\frac{\partial n}{\partial t} = \pi \int \vert T^{k k_1}_{k_2 k_3}\vert^2 \delta_{k+k_1-k_2-k_3} \delta_{\omega_{k}+\omega_{k_1}-\omega_{k_2}-\omega_{k_3}}\times \cr$$ (1.3)

This equation is exactly equivalent (see [4]) to the Hasselmann's equation, derived first in 1962 [5]. The equation (1.3) is entirely adequate to the situation if $\vec k_i$ are two-dimensional vectors. But it completely fails in the one-dimensional case.

The equations (1.1) have in one-dimensional case two types of solutions:

1. The trivial solutions:

$$k_2 = k_1,\hspace{.5cm} k_3 = k,\cr or\hspace{.5cm}k_3$$ (1.4)

Here $k$ and $k_1$ can have same or opposite signs.

2. The nontrivial solutions ( $k_2 \neq k, k_1$).

These solutions exist only if the products $k k_1$ and $k_2 k_3$ have opposite signs. They can be described analytically as follow. Let $k > 0$, $k_1 > 0$, $k_2 < 0$, $k_3 > 0$. Then

$$k = a(1+\zeta)^2, \hspace{0.5cm}k_1 = a(1+\zeta)^2 \zeta^2, \cr k_2$$ (1.5)

If one of the conditions (1.4) holds, the expression

$$n_{k_2}n_{k_3}(n_k + n_{k_1}) - n_k n_{k_1}(n_{k_2}n_{k_3})$$

is equal to zero. So, the trivial solutions do not put any contribution to the kinetic equation (1.3). It is irrelevant if all the wave numbers have the same sign (waves propagate in the same direction). But even for waves propagating in the opposite directions, four-wave interaction vanishes. As it was shown by Dyachenko and Zakharov [6], the coefficient $T^{k k_1}_{k_2 k_3}$ is identically equal to zero

$$T^{k k_1}_{k_2 k_3} \equiv 0$$

on the manifold (1.5).

This remarkable identity means that the system (1.2) is approximately integrable and kinetic equation appears for the next order only

$$\frac{\partial n}{\partial t} = st(n,n,n,n).$$

Here $st(n,n,n,n)$ is the collision term due to five-wave interaction, which are governed by the following resonant conditions

$$\omega_{k} + \omega_{k_1} + \omega_{k_2} = \omega_{k_3} + \omega_{k_4} \cr k + k_1 + k_2$$ (1.6)

A corresponding Hamiltonian has a form

$$\mbox{$\cal H$} = \int \omega_k a_k a_k^* dk +\cr$$

The expression $st(n,n,n,n)$ looks like that

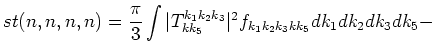$$st(n,n,n,n) = \frac{\pi}{3}\int \vert T^{k_1 k_2 k_3}_{k k_5}\vert^2 f_{k_1 k_2 k_3 k k_5}dk_1dk_2dk_3dk_5 - \cr$$ (1.7)

The expression (1.7) was found by Krasitskii [7]. He also found ``in principle'' the expression for $T^{k k_1 k_2}_{k_3 k_4}$. But his final formula is extraordinary complicated and cumbersome and hardly can be used for any practical purpose. He used a technique of the canonical transformation which exclude gradually the low order nonlinear terms in the Hamiltonian.

In this article we evaluate the coefficient $T^{k k_1 k_2}_{k_3 k_4}$ on the resonant surface (1.6). Our final formulae are astonishingly simple. This is one more miracle in the theory of surface waves. We also find the Kolmogorov's solution of the stationary equation

$$st(n,n,n,n) = 0$$

We will use a different technique then was used in [1],[2],[3],[5].