Example: Linear Schrödinger equation

Consider a one-dimensional example of a linear Schrödinger equation with a slowly varying potential. This equation is also referred to as linearized Gross-Pitaevsky equation. It is used to describe a formation of the BEC. It is given by

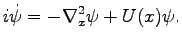$$i\dot\psi=-\nabla_{x}^2\psi+U(x)\psi.$$ (45)

This equation can be written in a Hamiltonian form with the Hamiltonian given by

$$H=\int\left(\vert\nabla_x\psi\vert^2+U(x)\vert\psi\vert^2\right)~dx$$

In the Fourier space, Eq. (49) becomes

$$i\frac{\partial}{\partial t}\hat{\psi}_k=k^2\hat{\psi}_k+\int\hat{U}(k-k_1)\hat{\psi}(k_1)~dk_1$$

with a corresponding Hamiltonian

$$H=\int\Omega(k,k_1)\hat{\psi}_k\hat{\psi}_{k_1}^*~dkdk_1,$$

where $\Omega(k,k_1)=k^2\delta^k_{k_1}+U(k_1-k)$ . Now we can apply Lemma and find that the position dependent dispersion becomes

$$\omega _{kx}=k^2+U(x)$$

and the corresponding Hamiltonian in terms of Gabor variables takes the canonical form (5):

$$H_f=\int \tilde{\psi}_{kx} [\omega_{kx}-x\nabla_x \omega_{kx}+i\{\omega_{kx},\cdot\}]\tilde{\psi}_{kx}^*dk dx.$$ (46)

It follows from the Lemma that the Gabor variables provide a canonical description of the system (49). Indeed, since $\nabla_k\nabla_x\omega _{kx}=0$ , we do not need to make a near-identity transformation (41) in the Lemma. Therefore, it is instructive to obtain the same result by directly applying Gabor transform to the both sides of Eq. (49). We have

$$\Gamma[\nabla_x^2\psi]=\nabla_x^2\tilde{\psi}_{kx}+ 2ik\nabla_x\t... ...-k^2\tilde{\psi}_{kx}\approx2ik\nabla_x\tilde{\psi}_{kx} -k^2\tilde{\psi}_{kx}.$$ (47)

To obtain this equation we have neglected the higher order derivative of the Gabor variable, since it is a slowly varying in $x$ . Next, we use the linear expansion of the potential $U(x_0)\approx U(x)+(x_0-x)\nabla_xU(x)$ to find

$$\Gamma[U(x)\psi]=(U(x)-x\nabla_xU(x))\tilde{\psi}_{kx}+ i\nabla_xU(x)\nabla_k\tilde{\psi}_{kx}$$ (48)

Combining Eq. (51) with Eq. (52), we obtain

$$i\frac{\partial}{\partial t}\tilde{\psi}_{kx}=(k^2+U(x)-x\nabla_x... ...\psi}_{kx}+ i\nabla_xU(x)\nabla_k\tilde{\psi}_{kx}-2ik\nabla_x\tilde{\psi}_{kx}$$

And the corresponding Hamiltonian is given by Eq. (50).