


Next: Evolution of statistics of
Up: Evolution of statistics of
Previous: Equation for the generating
Taking the inverse Laplace transform of (64) we have the following
equation for the PDF,
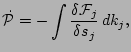 |
(59) |
where
 is a flux of probability in the space of the amplitude
is a flux of probability in the space of the amplitude  ,
,
This equation is identical to the one originally obtained
by Peierls [19] and later rediscovered
by Brout and Prigogine [20] in the context of
the physics of anharmonic crystals,
![$\displaystyle \dot {\cal P} = {16 \pi {\epsilon}^2 } \int \vert V_{mn}^{j}\vert...
...s_n \left[{\delta \over \delta s} \right]_3 {\cal P} \right) \, dk_j dk_m dk_n,$](img345.png) |
(61) |
where
![$\displaystyle \left[{\delta \over \delta s} \right]_3 = {\delta \over \delta s_j} - {\delta \over \delta s_m} -{\delta \over \delta s_n}.$](img346.png) |
(62) |
Zaslavski and Sagdeev [21] were the first to study this
equation in the WT context. However, the analysis of
[19,20,21]
was restricted
to the interaction Hamiltonians of the ``potential energy'' type,
i.e. the ones that involve only the coordinates but not the momenta.
This restriction leaves aside a great many important WT systems,
e.g. the capillary, Rossby, internal and MHD waves.
Our result above indicates that the Peierls equation
is also valid in the most general case of 3-wave systems.
Note that Peierls form (67) - (68) looks somewhat more
elegant and symmetric than (65) - (66).
However, form (65) - (66) has advantage because it is
in a continuity equation form. Particularly for steady state solutions, one
can immediately integrate it once and obtain,
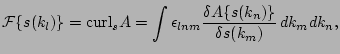 |
(63) |
where  is an arbitrary functional of
is an arbitrary functional of  and
and
 is the antisymmetric tensor,
is the antisymmetric tensor,
In the other words, probability flux  can be an arbitrary solenoidal field
in the functional space of
can be an arbitrary solenoidal field
in the functional space of  . One can see that (69) is a
first order equation with respect to the
. One can see that (69) is a
first order equation with respect to the  -derivative.
Special cases of the steady solutions are the zero-flux and the constant-flux
solutions which, as we will see later correspond to a Gaussian and
intermittent wave turbulence respectively.
-derivative.
Special cases of the steady solutions are the zero-flux and the constant-flux
solutions which, as we will see later correspond to a Gaussian and
intermittent wave turbulence respectively.
Here we should again emphasise importance of the taken order
of limits,
 first and
first and
 second.
Physically this means that the frequency resonance is broad
enough to cover great many modes. Some authors, e.g. [19,20,21],
leave the sum notation in the PDF equation even after the
second.
Physically this means that the frequency resonance is broad
enough to cover great many modes. Some authors, e.g. [19,20,21],
leave the sum notation in the PDF equation even after the
 limit taken giving
limit taken giving
 .
One has to be careful interpreting such formulae because
formally the RHS is nill in most of the cases because
there may be no exact resonances between the discrete
.
One has to be careful interpreting such formulae because
formally the RHS is nill in most of the cases because
there may be no exact resonances between the discrete  modes
(as it is the case, e.g. for the capillary waves). In real finite-size
physical systems, this condition means that the wave amplitudes, although
small, should not be too small so that the frequency broadening is sufficient
to allow the resonant interactions. Our
functional integral notation is meant to indicate that
the
modes
(as it is the case, e.g. for the capillary waves). In real finite-size
physical systems, this condition means that the wave amplitudes, although
small, should not be too small so that the frequency broadening is sufficient
to allow the resonant interactions. Our
functional integral notation is meant to indicate that
the
 limit has already been taken.
limit has already been taken.



Next: Evolution of statistics of
Up: Evolution of statistics of
Previous: Equation for the generating
Dr Yuri V Lvov
2007-01-23
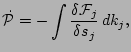
![]() first and
first and
![]() second.
Physically this means that the frequency resonance is broad
enough to cover great many modes. Some authors, e.g. [19,20,21],
leave the sum notation in the PDF equation even after the
second.
Physically this means that the frequency resonance is broad
enough to cover great many modes. Some authors, e.g. [19,20,21],
leave the sum notation in the PDF equation even after the
![]() limit taken giving
limit taken giving
![]() .
One has to be careful interpreting such formulae because
formally the RHS is nill in most of the cases because
there may be no exact resonances between the discrete
.
One has to be careful interpreting such formulae because
formally the RHS is nill in most of the cases because
there may be no exact resonances between the discrete ![]() modes
(as it is the case, e.g. for the capillary waves). In real finite-size
physical systems, this condition means that the wave amplitudes, although
small, should not be too small so that the frequency broadening is sufficient
to allow the resonant interactions. Our
functional integral notation is meant to indicate that
the
modes
(as it is the case, e.g. for the capillary waves). In real finite-size
physical systems, this condition means that the wave amplitudes, although
small, should not be too small so that the frequency broadening is sufficient
to allow the resonant interactions. Our
functional integral notation is meant to indicate that
the
![]() limit has already been taken.
limit has already been taken.