Weak nonlinearity expansion: three-wave case.

Substituting the expansion (32) in (12) we get in the zeroth order

$$a_l^{(0)}(T)=a_l(0),$$

i.e. the zeroth order term is time independent. This corresponds to the fact that the interaction representation wave amplitudes are constant in the linear approximation. For simplicity, we will write $a^{(0)}_l(0)= a_l$, understanding that a quantity is taken at $T=0$ if its time argument is not mentioned explicitly.

Here we have taken into account that $a^{(0)}_l(T)= a_l$ and $a^{(1)}_k (0)=0$.

The first order is given by

$$a^{(1)}_l (T) = -i \sum_{m,n=1}^\infty \left( V^l_{mn} a_m a_n \Delta^l_{mn} \delta^l_{m+n}\right.$$

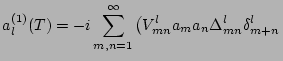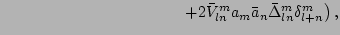$$\left.\hskip 4cm + 2 \bar{V}^m_{ln}a_m\bar{a}_n \bar\Delta^m_{ln}\delta^m_{l+n} \right),$$ (30)

Here we have taken into account that $a^{(0)}_l(T)= a_l$ and $a^{(1)}_k (0)=0$. Perform the second iteration, and integrate over time to obtain To calculate the second iterate, write

$$i\dot{ a}^{(2)}_l = \sum_{m,n=1}^\infty \Big[ V^l_{mn}\delta^l_{m+n} e^{i \omega^l_{mn} t}\left(a_m^{(0)} a_n^{(1)}+ a_m^{(1)} a_n^{(0)}\right)$$

$$\hspace{3cm}+2\bar{V}^m_{ln}\delta^m_{l+n} e^{ -i \omega^m_{ln} t}\left(a_m^{(1)} \bar{a}_n^{(0)}+ a_m^{(0)} \bar{a}_n^{(1)}\right)\Big].$$

Substitute (34) into (35) and integrate over time to obtain

$$a_l^{(2)} (T) = \sum_{m,n, \mu, \nu=1}^\infty \left[ 2 V^l_{mn} \left( -V^m_{\mu ... ...l \nu}_{n \mu},\omega^l_{mn}]\delta^\mu_{m + \nu}\right) \delta^l_{m+n} \right.$$

$$\left. + 2 \bar V^m_{ln} \left( -V^m_{\mu \nu}\bar a_n a_\mu a_\n... ...{n \nu l},-\omega^m_{l n}] \delta^\mu_{m + \nu} \right) \delta^m_{l+ n} \right.$$

$$\left. + 2 \bar V^m_{ln} \left( \bar V^n_{\mu \nu}a_m \bar a_\mu ... ...u l}_{\nu m}, -\omega^m_{ln}]\delta^\mu_{n + \nu}\right)\delta^m_{l+n} \right],$$

where we used $a^{(2)}_k (0)=0$. Hereafter, we drop super-script $(0)$ in expressions like (36) for brevity of notations.