Canonical variables and the Hamiltonian of the problem

The basic set of equations describing a two-dimensional potential flow of an ideal incompressible fluid with a free surface in a gravity field fluid is the following one:

$$\phi_{xx} + \phi_{zz} = 0 \hspace{1cm} (\phi_z\to 0, z\to -\infty), \cr \eta_t + \eta_x\phi_x$$

here $\eta(x,t)$ is the shape of a surface, $\phi(x,z,t)$ is a potential function of the flow and $g$ - is a gravitational constant. As was shown by Zakharov in[8], the potential on the surface $\psi(x,t) = \phi(x,z,t)\bigg\vert _{z=\eta}$ and $\eta(x,t)$ are canonically conjugated, and their Fourier transforms satisfy the equations

$$\frac{\partial \psi_k}{\partial t} = -\frac{\delta \mbox{$\ca... ...}{\partial t} = \frac{\delta \mbox{$\cal H$}}{\delta \psi_k^*}.$$

Here $\mbox{$\cal H$}=K+U$ is the total energy of the fluid with the following kinetic and potential energy terms:

$$K = \frac{1}{2}\int\!dx\!\int_{-\infty}^\eta v^2\!dz \hspace{1cm} U = \frac{g}{2}\int \eta^2\!dx$$

A Hamiltonian can be expanded in an infinite series in powers of a characteristic wave steepness $k\eta_k <\!< 1$ ([,]) by using an iterative procedure. All terms up to the fifth order of this series contribute to the amplitude of the five-wave interaction. So the Hamiltonian is expressed in terms of complex wave amplitudes $a_k, a_k^*$ which satisfies the canonical equation of motion:

$$\nonumber \frac{\partial a_k}{\partial t} + i\frac{\delta \mbox{$\cal H$}}{\delta a_k^*}=0.$$

here $\omega_k = \sqrt{g\vert k\vert}$ -is the dispersion law for the gravity waves. $\mbox{$\cal H$}$ can be expanded as follow

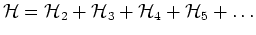$$\mbox{$\cal H$}= \mbox{$\cal H$}_2 + \mbox{$\cal H$}_3 + \mbox{$\cal H$}_4 + \mbox{$\cal H$}_5 + \ldots$$ (2.1)

In the normal variable $a_k$ the second order term in the Hamiltonian acquires the form:

$$\mbox{$\cal H$}_2 = \int \omega_k a_k a_k^* dk$$

The third order term, which describes $0\Leftrightarrow 3$ (first line) and $1\Leftrightarrow 2$ processes (second line) is:

$$\mbox{$\cal H$}_3 = \frac{1}{2}\int\!V^{k_1}_{k_2 k_3} \{a_{k_1}^* a_{k_2} a_{k_3}+a_{k_1} a_{k_2}^* a_{k_3}^*\} \delta_{k_1-k_2-k_3}\!dk_1dk_2dk_3+\cr$$

Fourth order term in the Hamiltonian consists of three terms:

$$\mbox{$\cal H$}_4 = \frac{1}{24}\int R_{k_1 k_2 k_3 k_4} (a_{k_1}a_{k_2}a_{k_3}a_{k_4}+a_{k_1}^*a_{k_2... ..._{k_4}^*)\times \delta_{k_1+k_2+k_3+k_4}\!dk_1dk_2dk_3dk_4\cr + \frac{1}{6}\int$$

describing different types of wave interactions (first line is ${4\Leftrightarrow 0}$, second line is ${3\Leftrightarrow 1}$, last line is ${2\Leftrightarrow 2}$ interactions).

Among the different terms of the fifth order, the only term corresponding to the process $2\Leftrightarrow3$ is considered:

$$\mbox{$\cal H$}_5 = \!\frac{1}{12}\int Q^{k_1 k_2 k_3}_{k_4 k_5} ... ...}^* a_{k_4} a_{k_5}+\!c.c.\} \delta_{k_1+k_2+k_3-k_4-k_5}\!dk_1dk_2dk_3dk_4dk_5$$

Here $V^{k_1}_{k_2,k_3}, U_{k_1,k_2,k_3},R_{k_1 k_2 k_3 k_4}, G^{k_1}_{k_2 k_3 k_4}, W^{k_1 k_2}_{k_3 k_4}, Q^{k_1 k_2 k_3}_{k_4 k_5}$ are interaction matrix elements of third, fourth and fifth order.

The Hamiltonian $\mbox{$\cal H$}$ in the normal variables $a_k$ is too complicated to work with. Our purpose is to simplify the Hamiltonian to the form:

$$ \mbox{$\cal H$} = \int \omega_k b_k b_k^* dk + \frac{1}{4}\int T^{k_1 k_2}_{k_3 k... ...{k_1}^*b_{k_2}^*b_{k_3}b_{k_4} \delta_{k_1+k_2-k_3-k_4}dk_1dk_2dk_3dk_4+ \cr \!$$ (2.2)

One of the ways to do that is to perform a canonical transformation [10], [11]

$$a_k = b_k + \int \Gamma^{k}_{k_1 k_2}b_{k_1}b_{k_2}\delta_{k-k_1-k_2} - 2\int \Gamma^{k_2}_{k k_1}b^*_{k_1}b_{k_2}\delta_{k+k_1-k_2} +\cr$$ (2.3)

where the $\Gamma$'s and $B$'s are determined in such a way that the transformation is canonical, and that the transformed Hamiltonian has the form (2.3). The transformation (2.4) is canonical up to the terms of order of $\vert b_k\vert^3$.

On the resonant manifold $\omega_{k} + \omega_{k_1} = \omega_{k_2} + \omega_{k_3} \ \vec k + \vec k_1 = \vec k_2 + \vec k_3$ there are two types of resonances - trivial and nontrivial. Trivial resonances are $k_2 = k_1,\hspace{.5cm} k_3 = k, or\hspace{.5cm}k_3 = k_1,\hspace{.5cm} k_2=k$. Nontrivial resonances may be parameterized as

$$k = a(1+\zeta)^2, \hspace{0.5cm}k_1 = a(1+\zeta)^2 \zeta^2, \cr k_2$$ (2.4)

It was shown in [2],[5,6] that on the nontrivial manifold (2.5) $T^{k,k_1}_{k_2,k_3}\equiv 0$, i.e. four-wave processes do not produce ``new wave vectors'', and that system is integrable to this degree of accuracy. This was the main motivation for investigating fifth order interactions.

To find $T^{k k_1 k_2}_{k_3 k_4}$ one can calculate the terms of the order of $b^3$ and $b^4$ in the canonical transformation (2.4). This very cumbersome procedure was fulfilled by V.Krasitskii[12], but the resulting expressions are so complicated that they can hardly be used for any practical purpose. Here the method of Feinman diagrams presented in [13], [1] is used.

First one introduce the so called formal classical scattering matrix which relates the asymptotic states of the system ``before'' and ``after'' interactions:

$$c^{+}_k = \hat S[c^{-}_k]$$

By ``for $\hat S[c^{-}_k]$ is a nonlinear operator which can be presented as a series in powers of $c^{-}, {c^{-}}^{*}$. It has the following form

$$\hat S[c^{-}_k] = c^{-}_k - \sum_{n+m\ge3}^{} \frac{2\pi i}{(n-1)!m!}\int S_{n m}(k,k_1,\ldots,k_{n-1};k_n,\ldots,k_{n+m-1})\times\cr$$ (2.5)

We will treat this series as formal one and will not care about their convergence [1,14]. The functions $S_{n m}$ are the elements of the scattering matrix. They are defined on the resonant manifolds

$$\vec k+\vec k_1+\ldots +\vec k_{n-1} = \vec k_n+\ldots +\vec k_{n+m-1} \cr \omega_k+\omega_{k_1}+\ldots +\omega_{k_{n-1}}$$ (2.6)

Note, that the value of the matrix element $S_{n m}$ on the resonant manifold (2.7) is invariant with respect to the canonical transformation (2.4) and that there is a simple algorithm for calculation of the matrix elements. The element $S_{n m}$ is a finite sum of the terms which can be expressed through the coefficients of the Hamiltonians $H_i, i\le {n+m}$. Each term can be marked by a certain Feinman diagram taken in a "tree" approximation, i.e. having no internal loops. To calculate $T^{k k_1 k_2}_{k_3 k_4}$ one calculates the first nonzero elements of the scattering matrix for the Hamiltonian (2.2) and for the Hamiltonian (2.3). Because these two Hamiltonians are connected by the canonical transformation (2.4), the results must coincide. The first nontrivial element of the scattering matrix in the one-dimensional case is

$$S_{3 2}(k,k_1,k_2,k_3,k_4) = T^{k k_1 k_2}_{k_3 k_4}$$

If $S_{3 2}(k,k_1,k_2,k_3,k_4)$ is calculated in terms of the initial Hamiltonian (2.2) it consists of 81 terms, with 60 diagrams combining three third order interactions (one of such diagrams with the corresponding expressions is shown below):

59#1

60#2

and also 20 diagrams combining third- and fourth order interactions (one of such diagrams with the corresponding expressions is shown below):

61#3

62#4

and also the fifth order vertex itself.

We use "Mathematica 2.2" for performing the analytical and numerical calculations of this paper. Initially, the expression for 63#5 occupies 1 Megabyte of computer memory, but we were able to simplify it to the form presented below. For some of the orientations, 64#6 is equal to zero. We verify this fact by computing 65#7 numerically on 100 random points of the resonant manifold (3.8) and get zero with accuracy of 66#8.