Five-wave kinetic equation and its solutions

The matrix element $T^{k k_1}_{k_2 k_3 k_4}$ for $3\Leftrightarrow 2$ process along with $T^{k k_1}_{k_2 k_3}$ allows us to derive kinetic equation which includes four- and five-wave interactions.

The dynamical equation for $b_k$ (with the Hamiltonian (4.1)) is:

$$\frac{\partial b_k}{\partial t} + i\omega_k b_k + i\frac{1}{2}\int T^{k k_1}_{k_2 k_3} b^*_{k_1} b_{k_2} b_{k_3} \delta_{k+k_1-k_2-k_3}dk_1dk_2dk_3 + \cr$$ (6.1)

Introducing standard pair correlation function $n_k$

$$<b_k b_{k_1}> = n_k \delta_{k-k_1}$$

we can derive from (6.1) the equation for $n_k$:

$$\frac{\partial n_k}{\partial t} = {\tt Im} \int T^{k k_1}_{k_2 k_3}<b^*_{k}b^*_{k_1}b_{k_2}b_{k_3}> \delta_{k+k_1-k_2-k_4}dk_1dk_2dk_3 + \cr$$

It is obvious that $T^{k k_1}_{k_2 k_3}$ contributes to the equation for fourth-order correlator, while $T^{k_1 k_2 k_3}_{k_4 k_5}$ contributes to the equation for fifth-order correlator only (due to the seventh-order correlators vanish). Fifth-order correlation function $<b^*_{k_1}b^*_{k_2}b^*_{k_3}b_{k_4}b_{k_5}>$ can be expressed through the eighth-order correlator:

$$(\frac{\partial }{\partial t}- i(\omega_{k_1}+\omega_{k_2}+\omega... ...}-\omega_{k_4}-\omega_{k_5})) <b^*_{k_1}b^*_{k_2}b^*_{k_3}b_{k_4}b_{k_5}> = \cr$$

Applying random phase approximation for the eighth-order correlator (to split it in a product of pair correlation functions) and assuming slow variation in time for fifth-order correlator, one can get the following expression for $<b^*_{k_1}b^*_{k_2}b^*_{k_3}b_{k_4}b_{k_5}>$:

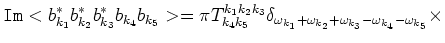$${\tt Im}<b^*_{k_1}b^*_{k_2}b^*_{k_3}b_{k_4}b_{k_5}> = \pi T^{k_1 ... ...a_{\omega_{k_1}+\omega_{k_2}+\omega_{k_3}- \omega_{k_4}-\omega_{k_5}}\times \cr$$ (6.2)

In the (6.2) we drop the terms which are out of resonant surface. For fourth-order correlator we have the following equaton [15]:

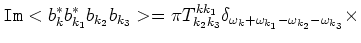$${\tt Im}<b^*_{k}b^*_{k_1}b_{k_2}b_{k_3}> = \pi T^{k k_1}_{k_2 k_3} \delta_{\omega_{k}+\omega_{k_1}-\omega_{k_2}-\omega_{k_3}}\times \cr$$ (6.3)

Substituting (6.3) and (6.2) into the equation for $n_k$ we get five-wave kinetic equation:

$$\frac{\partial n_k}{\partial t} = st(n,n,n) + st(n,n,n,n)$$

$$st(n,n,n) = \pi\int \vert T^{k k_1}_{k_2 k_3}\vert^2 f^{k k_1}_{k_2 k_3}dk_1dk_2dk_3\cr st(n,n,n,n)$$

$$f^{k k_1}_{k_2 k_3} = \delta_{k+k_1-k_2-k_3} \delta_{\omega_{k}+\omega_{k_1} -\omega_{k_2}-\omega_{k_3}}\times \cr$$

As it was shown in the Introction, In the one dimensional case $st(n,n,n)\equiv0$ and we end up with the pure five-wave kinetic equation:

$$\frac{\partial n_k}{\partial t} = st(n,n,n,n)$$ (6.4)

The equation (6.4) formally preserves two integrals of motion, energy

$$E = \int_{0}^{\infty} \omega_{k}n_k dk,$$

and momentum

$$P = \int_{0}^{\infty} k n_k dk$$

(We consider the case when all $k_i$ are positive). The stationary equation

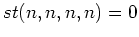$$st(n,n,n,n) = 0$$ (6.5)

has thermodynamic solution

$$n_k = \frac{T}{\omega_{k}+\alpha k}.$$

Like four-wave isotropic kinetic equation, the equation (6.4) describes direct and inverse cascades. The inverse cascade is the cascade of energy, which is a real constant of motion and is carried toward the small $k$. It is described by the following Kolmogorov solution of the equation (6.5)

$$n^{(1)}_k = \alpha^{(1)} \epsilon^{1/4} \vert k\vert^{-25/8}$$

Here $\epsilon$ is the energy flux, $\alpha^{(1)}$ is the Kolmogorov constant.

A corresponding energy spectrum is

$$\cal E_{\omega}d\omega = \omega_k n_k dk\cr \cal E_{\omega}$$

Direct cascade is a transport of momentum towards the large wave numbers. It is described by the Kolmogorov solution

$$n^{(2)}_k = \alpha^{(2)} \mu^{1/4} \vert k\vert^{-13/4}$$

Here $\mu$ is the momentum flux, $\alpha^{(2)}$ is the Kolmogorov constant.

Now

$$\cal E_{\omega} = \alpha^{(2)} \mu^{1/4}{\omega}^{-9/2}$$

Due to the direct cascade the momentum is not a real constant of motion, it leaks permanently to the large $k$ region. More detailed description of the Kolmogorov spectra in one-dimensional case will be published separately.

In conclusion authors express gratitude to Dr. Victor L'vov for his valuable advises and to Alina Spectorov for preparing the diagrams.

This work is supported by the ONR Grant N00 14-92-J-1343 and Russian Basic Research Foundation Grant N00 94-01-00898. Y.L. acknowledges support from AFOSR Grant F49620-94-1-0144DEF.