


Next: Preservation of the RPA
Up: Theoretical WT predictions
Previous: Theoretical WT predictions
In [5] the following
equation for
for the  -mode PDF was obtained for the four-wave systems,
-mode PDF was obtained for the four-wave systems,
![$\displaystyle \dot {\cal P} = { \pi {\epsilon}^2 } \int \vert W^{jl}_{nm}\vert^...
...a s} \right]_4 {\cal P} \right) \, d{\bf k}_j d{\bf k}_l d{\bf k}_m d{\bf k}_n,$](img85.png) |
(16) |
where
![$\displaystyle \left[{\delta \over \delta s} \right]_4 = {\delta \over \delta s_...
...delta \over \delta s_l} - {\delta \over \delta s_m} -{\delta \over \delta s_n}.$](img86.png) |
(17) |
Here
 limit has already been taken and
limit has already been taken and
 means the variational derivative. Using this
equation, one can prove that RPA property holds over the nonlinear
time, i.e. the
means the variational derivative. Using this
equation, one can prove that RPA property holds over the nonlinear
time, i.e. the  -mode PDF remains of the product factorized form
with accuracy sufficient for the WT closures to work [5].
Using RPA, we get for the one-point marginals [5],
-mode PDF remains of the product factorized form
with accuracy sufficient for the WT closures to work [5].
Using RPA, we get for the one-point marginals [5],
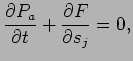 |
(18) |
with  is a probability flux in the s-space,
is a probability flux in the s-space,
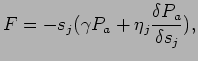 |
(19) |
where
Here we introduced the wave-action spectrum,
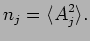 |
(22) |
From (14) we get the following equation
for the moments
 :
:
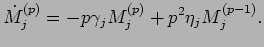 |
(23) |
which, for  gives the standard wave kinetic equation (WKE),
gives the standard wave kinetic equation (WKE),
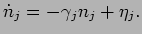 |
(24) |



Next: Preservation of the RPA
Up: Theoretical WT predictions
Previous: Theoretical WT predictions
Dr Yuri V Lvov
2007-01-16
![]() -mode PDF was obtained for the four-wave systems,
-mode PDF was obtained for the four-wave systems,