


Next: Phase statistics.
Up: Joint statistics of amplitudes
Previous: Approximate independence of the
We have established above that
the one-point statistics is at the heart of the WT theory.
All one-point statistical objects can be derived from the one-point
amplitude generating function,
which can be obtained from the  -point
-point  by taking all
by taking all  's
and all
's
and all  's, except for
's, except for  , equal to zero.
Substituting such values to (35) we get the following
equation for
, equal to zero.
Substituting such values to (35) we get the following
equation for  ,
,
 |
(42) |
where,
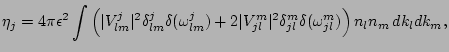 |
|
|
(43) |
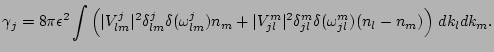 |
|
|
(44) |
Correspondingly, for the one mode PDF  we have
we have
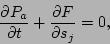 |
(45) |
with  is a probability flux in the s-space,
is a probability flux in the s-space,
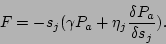 |
(46) |
Equations (43) and (46) where previously obtained and
studied in [20] in for the four-wave systems.
The only difference for the four-wave case was different
expressions for  and
and  . For the three-wave case,
equation for the PDF was not considered before, but equations
for its moments were derived and solved in [19].
In particular, equation for the first moment is nothing but the
familiar kinetic equation
. For the three-wave case,
equation for the PDF was not considered before, but equations
for its moments were derived and solved in [19].
In particular, equation for the first moment is nothing but the
familiar kinetic equation
 which
gives
which
gives
 for any steady state. This, in turn means
that in the steady state with
for any steady state. This, in turn means
that in the steady state with  we have
we have
 where
where  can be any steady state
solution of th kinetic equation including the KZ spectrum which plays
the central role in WT [5,1].
However, it was shown in [20] that there also exist
solutions with
can be any steady state
solution of th kinetic equation including the KZ spectrum which plays
the central role in WT [5,1].
However, it was shown in [20] that there also exist
solutions with  which describe WT intermittency.
which describe WT intermittency.



Next: Phase statistics.
Up: Joint statistics of amplitudes
Previous: Approximate independence of the
Dr Yuri V Lvov
2007-01-17